Math Lesson 10.2.3 - Solving Quadratic Inequalities by Studying the Sign

Please provide a rating, it takes seconds and helps us to keep this resource free for all to use

Welcome to our Math lesson on Solving Quadratic Inequalities by Studying the Sign, this is the third lesson of our suite of math lessons covering the topic of Quadratic Inequalities, you can find links to the other lessons within this tutorial and access additional Math learning resources below this lesson.

Solving Quadratic Inequalities by Studying the Sign

Obviously, it is not possible to find the solution set of a quadratic inequality simply by guessing or trying all values that come to mind. Therefore, we must find a general method for solving such inequalities. This method consists of studying the sign of inequality. For this, we also have to take the corresponding quadratic equation into account which allows us to determine the boundary values where the sign of the inequality changes.

There are three cases to consider when solving a quadratic equation by studying the sign:

1. The discriminant of the corresponding quadratic equation is positive

Whenever we have a quadratic inequality, we must first check the solutions (roots) of the corresponding quadratic equation. Thus, if we have for example the quadratic inequality

we must first check for the number of solutions of the corresponding quadratic equation

by finding the value of the discriminant Δ. From tutorial 9.6, we know that if the discriminant Δ is positive, the quadratic equation has two distinct roots, if Δ = 0, the equation has a single root (in fact, two equal roots) and if Δ < 0, the equation has no roots. This is because the square root of a negative number does not exist in R.

The quadratic formula that allows us to solve all quadratic equations is

where

The first rule we use to solve a linear inequality by studying the sign is as follows:

"If the discriminant of the corresponding quadratic equation is positive, the quadratic inequality has the opposite sign of the constant a between the roots and the sign of the coefficient a aside from the interval between the two roots."

This rule is illustrated in the scheme below.

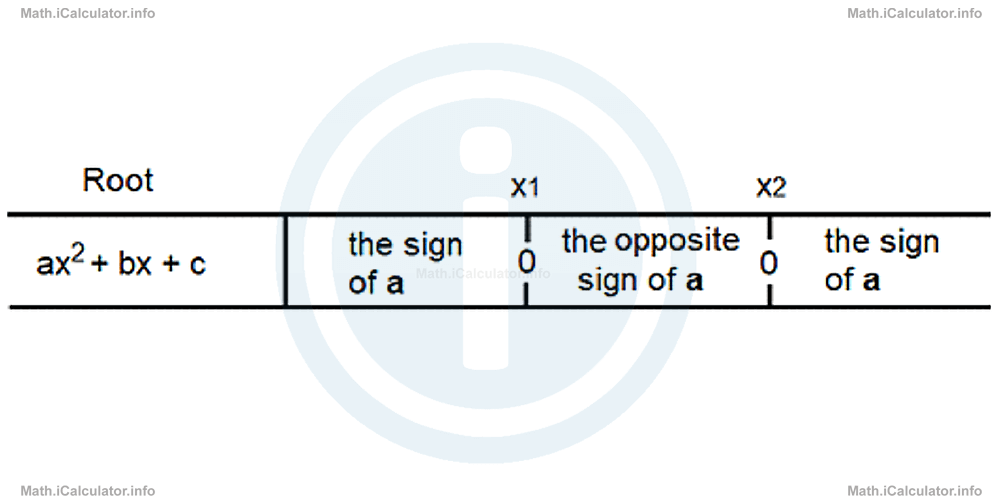
For example, in the quadratic inequality

we have a = 1, b = -3 and c = 2. Thus, the discriminant Δ is

Since Δ > 0, the corresponding equation has two roots. They are

and

Hence, since a > 0 (a = 1), the original quadratic inequality is negative between 1 and 2 and positive outside this range. We need it to be negative or zero. Therefore, the solution set of the inequality

is the segment [1, 2].

We can prove this solution is corect by picking a number inside the solution set and checking whether that number gives a true result. Let's take for example x = 1.5. Thus,

On the other hand, for values of x outside the solution range, for example for x = 0, which is a value below the solution range, we have

and for x = 3, which is a value above the solution range, we have

Hence, the correctness of our solution and of the method used for solving quadratic inequalities is confirmed.

Example 3

Find the solution set of the quadratic inequalities

1. 3 - 2x < x²
2. 2x² - 5x < -3

Solution 3

1. First, we have to send all terms on the right side. Thus,
   3 - 2x < x²
   -x² - 2x + 3<0
   (-1) ∙ (-x² - 2x + 3) < (-1) ∙ 0
   x² + 2x - 3 > 0
   Now, let's study the sign of this inequality by considering the corresponding quadratic equation
   x² + 2x - 3 = 0
   We have a = 1, b = 2 and c = -3. Thus,
   ∆ = b² - 4ac
   = 2² - 4 ∙ 1 ∙ (-3)
   = 4 + 12
   = 16
   Given that the discriminant is positive, the two roots of the corresponding equation are
   x₁ = -b - √∆/2a
   = -2 - √16/2 ∙ 1
   = -2 - 4/2
   = -3
   and
   x₂ = -b + √∆/2a
   = -2 + √16/2 ∙ 1
   = -2 + 4/2
   = 1
   This means that in the interval (-3, 1), the quadratic expression is negative, as it has the opposite sign to the coefficient a. However, we are interested in the positive part of the expression, so the solution sets of our inequality extend outside the interval determined by the two roots. Hence, we have two solutions sets for this inequality, as shown below.
   x ϵ (-∞,-1) and x ϵ (3, + ∞)
2. Again, let's send everything to the left side first. Thus,
   2x² - 5x < - 3
   2x² - 5x + 3 < -3 + 3
   2x² - 5x + 3 < 0
   Now, let's study the sign of this inequality by considering the corresponding quadratic equation
   2x² - 5x + 3 = 0
   We have a = 2, b = -5 and c = 3. Thus,
   ∆ = b² - 4ac
   =(-5)² - 4 ∙ 2 ∙ 3
   =25 - 24
   =1
   Given that the discriminant is positive, the two roots of the corresponding equation are
   x₁ = -b - √∆/2a
   = -(-5) - √1/2 ∙ 2
   = 5 - 1/4
   = 1
   and
   x₂ = -b + √∆/2a
   = -(-5) + √1/2 ∙ 2
   = 5 + 1/4
   = 6/4
   = 3/2
   This means that in the interval (-3, 1), the quadratic expression is negative, as it has the opposite sign to the coefficient a. We are interested precisely in this interval, as the inequality bears the "smaller than" symbol, which means it must be negative to be true. Hence, the solution set for this inequality is
   x ϵ (1,3/2)

2. The discriminant of the corresponding quadratic equation is zero

In this case, the interval between the two roots in the previous case narrows and becomes zero, as the two roots converge. Therefore, we have only one zero point where the quadratic inequality turns into a quadratic equation, while for the rest of the values the quadratic expression has the sign of the coefficient a, as shown in the scheme below.

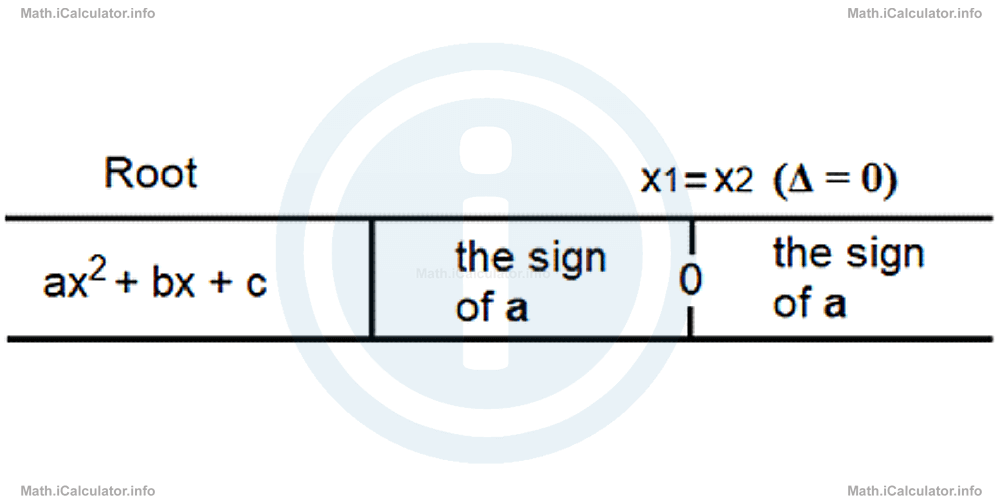
For example, the inequality

is always true because it has a single root where it becomes zero and everywhere else it is negative, as it has the opposite sign of the coefficient a (a = 2).

Indeed, we have a = 2, b = -8 and c = 8. Thus, the discriminant Δ is

Hence, since √Δ = √0 = 0, we obtain

If the sign in the original inequality was simply " < " instead of " ≤ ", we would have two intervals as solution sets that include all real values except 2. We write these solutions sets as

Example 4

Solve the following inequalities.

1. x² + 6x + 9 > 0
2. x² - 8x + 16 ≤ 0

Solution 4

1. Let's consider the corresponding quadratic equation
   x² + 6x + 9 = 0
   We have a = 1, b = 6 and c = 9. The discriminant Δ is
   ∆ = b² - 4ac
   = 6² - 4 ∙ 1 ∙ 9
   = 36 - 36
   = 0
   Hence, the double root of this equation is
   x₁ = x₂ = -b/2a
   = -6/2 ∙ 1
   = -6/2
   = -3
   This means we can write this quadratic equation in the factorised form as
   [x - (-3)]² = 0
   or
   (x + 3)² = 0
   We want the expression in the brackets to be positive, i.e
   (x + 3)² > 0
   This inequality is true for all values of x except -3. Indeed, from theory, we know that the sign of inequality aside from the roots is the same as the sign of a, which here is positive. Hence, the solution sets of this equality are
   x ϵ (-∞,-3) and x ϵ (-3, + ∞)
2. Again, let's consider the corresponding quadratic equation
   x² - 8x + 16 = 0
   We have a = 1, b = -8 and c = 16. The discriminant Δ is
   ∆=b² - 4ac
   =(-8)² - 4 ∙ 1 ∙ 16
   =64 - 64
   =0
   Hence, the double root of this equation is
   x₁ = x₂ = -b/2a
   = -(-8)/2 ∙ 1
   = 8/2
   = 4
   This means we can write this quadratic equation in the factorised form as
   (x - 4)² = 0
   We want the expression in the brackets to be negative or zero, i.e.
   (x - 4)² ≤ 0
   From the properties of indices, it is known that a number or expression raised to the second power cannot be negative. Therefore, the only value that makes our inequality true is x = 4, as for this value of the variable x the inequality becomes zero. Hence, the solution set of this inequality contains only one value: x = 4.

3. The discriminant of the corresponding quadratic equation is negative

In this case, the bordering value that separates the two intervals where the zone taking the sign of the coefficient a does not exist anymore, as the corresponding quadratic equation cannot have any solution as in the previous two cases. It is these solution points that made the separation between the zones possible. Therefore, the quadratic inequalities always have the sign of the coefficient a, as shown in the scheme below.

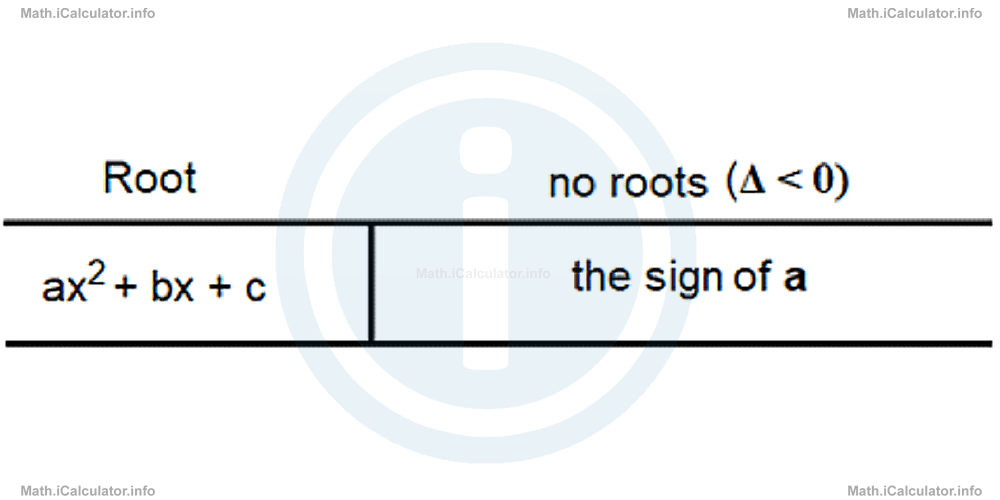
For example, the quadratic inequality 2x² - 5x + 4 > 0 is always true for all values of x. This is because a = 2, b = -5 and c = 4 and when calculating the discriminant Δ, we obtain

Therefore, the corresponding equation 2x² - 5x + 4 = 0 has no roots. This means the inequality 2x² - 5x + 4 > 0 is always true. Indeed, the expression on the left side of the inequality is always positive because the sign of a occurs everywhere.

On the other hand, the inequality 3x² + 4x + 2 ≤ 0 is always false for all values of x. This is because the discriminant is negative and therefore, the inequality always uses the sign of a (a = 2, so it is positive). Indeed, since a = 3, b = 4 and c = 2, we have

Example 5

Solve the following inequalities.

1. x² + 2x + 7 ≤ 0
2. 5x² - 3x + 1 > 0

Solution 5

More Quadratic Inequalities Lessons and Learning Resources

Whats next?

Enjoy the "Solving Quadratic Inequalities by Studying the Sign" math lesson? People who liked the "Quadratic Inequalities lesson found the following resources useful:

1. Sign Feedback. Helps other - Leave a rating for this sign (see below)
2. Inequalities Math tutorial: Quadratic Inequalities. Read the Quadratic Inequalities math tutorial and build your math knowledge of Inequalities
3. Inequalities Video tutorial: Quadratic Inequalities. Watch or listen to the Quadratic Inequalities video tutorial, a useful way to help you revise when travelling to and from school/college
4. Inequalities Revision Notes: Quadratic Inequalities. Print the notes so you can revise the key points covered in the math tutorial for Quadratic Inequalities
5. Inequalities Practice Questions: Quadratic Inequalities. Test and improve your knowledge of Quadratic Inequalities with example questins and answers
6. Check your calculations for Inequalities questions with our excellent Inequalities calculators which contain full equations and calculations clearly displayed line by line. See the Inequalities Calculators by iCalculator™ below.
7. Continuing learning inequalities - read our next math tutorial: Graphing Inequalities

Help others Learning Math just like you

Please provide a rating, it takes seconds and helps us to keep this resource free for all to use

We hope you found this Math tutorial "Quadratic Inequalities" useful. If you did it would be great if you could spare the time to rate this math tutorial (simply click on the number of stars that match your assessment of this math learning aide) and/or share on social media, this helps us identify popular tutorials and calculators and expand our free learning resources to support our users around the world have free access to expand their knowledge of math and other disciplines.

Inequalities Calculators by iCalculator™

• Quadratic Inequalities Calculator