Menu
Quadratic Inequalities Calculator
Please provide a rating, it takes seconds and helps us to keep this resource free for all to use
The Quadratic Inequalities Calculator will calculate:
- The range of the variable's values in any quadratic inequality when the coefficients a and b and the constant c are known.
Quadratic Inequalities Calculator Parameters: Calculating the root(s) of the given quadratic equation through the quadratic formula.
| First root of the quadratic equation (x1) = |
| Second root of the quadratic equation (x2) = |
| The solution set of the given inequality (x) = |
| First root of the quadratic equation Formula and Calculations |
|---|
| x1 = -b - √b2 - 4ac/2a x1 = - √2 - (4 × × )/2 × x1 = - √ - / x1 = - √/ x1 = - / x1 = / x1 = |
| Second root of the quadratic equation Formula and Calculations |
| x2 = -b + √b2 - 4ac/2a x2 = + √2 - (4 × × )/2 × x2 = + √ - / x2 = + √/ x2 = + / x2 = / x2 = |
| Quadratic Inequalities Calculator Input Values |
| Coefficient (a) = |
| Coefficient (b) = |
| Constant (c) = |
| Inequality sign is |
Please note that the formula for each calculation along with detailed calculations is shown further below this page. As you enter the specific factors of each quadratic inequalities calculation, the Quadratic Inequalities Calculator will automatically calculate the results and update the formula elements with each element of the quadratic inequalities calculation. You can then email or print this quadratic inequalities calculation as required for later use.
We hope you found the Quadratic Inequalities Calculator useful, if you did, we kindly request that you rate this calculator and, if you have time, share to your favourite social network. This allows us to allocate future resource and keep these Math calculators and educational material free for all to use across the globe.
Please provide a rating, it takes seconds and helps us to keep this resource free for all to use
Related Fields with Tutorials
Theoretical description
A quadratic inequality is a mathematical sentence of the form
where (?) represents one of the four inequality symbols ">", "<", "≥" and "≤".
On the other hand, a and b are coefficients, c is a constant and x is the only variable.
The easiest method for solving quadratic inequalities is to study the sign of the discriminant Δ. From quadratic equations, it is known that the formula for finding the discriminant is
Depending on the value of the discriminant, we can distinguish three different situations regarding the solution of quadratic inequalities.
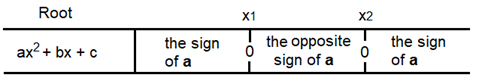
If the discriminant Δ is positive, there are two values for which the quadratic expression becomes zero. They are the two distinct roots of the corresponding quadratic equation calculated by the formulas
and
The sign of the inequality, in this case, obeys the following rule:
The quadratic inequality has the opposite sign of the coefficient 'a' between the two roots and the sign of 'a' outside this range. Only for values of the variable equal to the roots of the corresponding equation, this inequality becomes zero.
The table below is a good illustration of the above rule.
If the discriminant Δ is zero, there is a single value for which the quadratic expression becomes zero. This is the only root (otherwise known as the double root) of the corresponding quadratic equation calculated by the formula
This is because
The sign of the inequality, in this case, obeys the following rule:
The quadratic inequality has the sign of the coefficient 'a' for all values of the variable x. Only for the value of the variable that is equal to the root of the corresponding equation, this inequality becomes zero.
The table below is a good illustration of the above rule.

If the discriminant Δ is negative, there is no value for which the quadratic expression becomes zero. This is because the square root of negative numbers does not exist in the set of real numbers.
The sign of the inequality, in this case, obeys the following rule:
The quadratic inequality has the sign of the coefficient 'a' for all values of the variable x.
The table below is a good illustration of the above rule.

For example, in the quadratic inequality
first, we calculate the value of the discriminant Δ (given that a = 2, b = -3 and c = 1). Thus,
= (-3)2 - 4 ∙ 2 ∙ 1
= 9 - 8
= 1
Since the discriminant is positive, the corresponding quadratic equation has two distinct roots. They are
= - (-3) - √1/2 ∙ 2
= 3 - 1/4
= 2/4
= 1/2
and
= -(-3) + √1/2 ∙ 2
= 3 + 1/4
= 4/4
= 1
Therefore, between 1/2 and 1 the quadratic expression has a negative value (the opposite sign of the coefficient 'a') given that a = 2 (positive). On the other hand, for x < 1/2 and for x > 1 the quadratic expression is positive (it has the same sign as the coefficient 'a').
Given that the inequality requires the quadratic expression to be negative or zero, its solution set is the segment [1/2, 1].
As for the quadratic inequality
first, we calculate the value of the discriminant Δ (given that a = 1, b = -4 and c = 4),
= (-4)2 - 4 ∙ 1 ∙ 4
= 16 - 16
= 0
then, we find the only root of the corresponding quadratic equation through the formula
= -(-4)/2 ∙ 1
= 4/2
= 2
Therefore, for any value different from 2, the inequality is positive (it has the sign of 'a') and only for x = 2 it becomes zero.
The question requires the variable to be negative or zero. Therefore, the only solution for this inequality is x = 2.
Last, let's consider an example of a quadratic inequality where the discriminant is negative. For example, in the inequality
we have a = 3, b = -5 and c = 4. Therefore, the discriminant Δ is
= (-5)2 - 4 ∙ 3 ∙ 4
= 25 - 48
= -23
Hence, since the discriminant is negative, the corresponding quadratic equation has no roots. This means the inequality has everywhere the sign of the constant 'a' (here, positive). Therefore, the inequality is true for every value of the variable x.
Our calculator will help you identify automatically the solution set of any quadratic inequality if you insert the coefficients a and b, the constant c and the inequality sign involved in it. In this way, you will save precious time, as there is no need to calculate the discriminant, etc.
Inequalities Math Tutorials associated with the Quadratic Inequalities Calculator
The following Math tutorials are provided within the Inequalities section of our Free Math Tutorials. Each Inequalities tutorial includes detailed Inequalities formula and example of how to calculate and resolve specific Inequalities questions and problems. At the end of each Inequalities tutorial you will find Inequalities revision questions with a hidden answer that reveal when clicked. This allows you to learn about Inequalities and test your knowledge of Math by answering the revision questions on Inequalities.